Archive
2023 in the programming language standards’ world
Two weeks ago I was on a virtual meeting of IST/5, the committee responsible for programming language standards in the UK. IST/5 has a new chairman, Guy Davidson, whose efficiency is very unstandard’s like.
It’s been 18 months since I last reported on the programming language standards’ world, what has been going on?
2023 is going to be a bumper year for the publication of revised Standards of long-established programming language: COBOL, Fortran, C, and C++ (a revised Standard for Ada was published last year).
Yes, COBOL; a new COBOL Standard was published in January. Reports of its death were premature, e.g., my 2014 post suggesting that the latest version would be the last version of the Standard, and the closing of PL22.4, the US Cobol group, in 2017. There has even been progress on the COBOL front end for gcc, which now supports COBOL 85.
The size of the COBOL Standard has leapt from 955 to 1,229 pages (around new 200 pages in the normative text, 100 in the annexes). Comparing the 2014/2023 documents, I could not see any major additions, just lots of small changes spread throughout the document.
Every Standard has a project editor, the person tasked with creating a document that reflects the wishes/votes of its committee; the project editor sends the agreed upon document to ISO to be published as the official ISO Standard. The ISO editors would invariably request that the project editor make tiresome organizational changes to the document, and then add a front page and ISO copyright notice; from time to time an ISO editor took it upon themselves to reformat a document, sometimes completely mangling its contents. The latest diktat from ISO requires that submitted documents use the Cambria font. Why Cambria? What else other than it is the font used by the Microsoft Word template promoted by ISO as the standard format for Standard’s documents.
All project editors have stories to tell about shepherding their document through the ISO editing process. With three Standards (COBOL lives in a disjoint ecosystem) up for publication this year, ISO editorial issues have become a widespread topic of discussion in the bubble that is language standards.
Traditionally, anybody wanting to be actively involved with a language standard in the UK had to find the contact details of the convenor of the corresponding language panel, and then ask to be added to the panel mailing list. My, and others, understanding was that provided the person was a UK citizen or worked for a UK domiciled company, their application could not be turned down (not that people were/are banging on the door to join). BSI have slowly been computerizing everything, and, as of a few years ago, people can apply to join a panel via a web page; panel members are emailed the CV of applicants and asked if “… applicant’s knowledge would be beneficial to the work programme and panel…”. In the US, people pay an annual fee for membership of a language committee ($1,340/$2,275). Nobody seems to have asked whether the criteria for being accepted as a panel member has changed. Given that BSI had recently rejected somebodies application to join the C++ panel, the C++ panel convenor accepted the action to find out if the rules have changed.
In December, BSI emailed language panel members asking them to confirm that they were actively participating. One outcome of this review of active panel membership was the disbanding of panels with ‘few’ active members (‘few’ might be one or two, IST/5 members were not sure). The panels that I know to have survived this cull are: Fortran, C, Ada, and C++. I did not receive any email relating to two panels that I thought I was a member of; one or more panel convenors may be appealing their panel being culled.
Some language panels have been moribund for years, being little more than bullet points on the IST/5 agenda (those involved having retired or otherwise moved on).
Small team estimating in story points; a project dataset
Just before the end of last year a regular reader, Mr A., emailed to ask if I would be interested in analysing the software project data for the company he worked for. The wishing to be anonymous company sold physical products, and bespoke software that supported the business was written by a team of three.
Two factors made this dataset interesting, 1) it was for a small team, 2) story points were used for estimating tasks and actual time was recorded (I had not seen such data before).
There are probably hundreds of thousands of small software teams working in companies whose main line of business is far removed from software (a significant percentage of developers work within a small team supporting the activities of non-software companies; I cannot find the bls page listing developer employment across industry codes). These small teams are rarely studied. Software engineering research usually focuses on the practices of software based companies, or large software development projects, i.e., groups likely to be easily visible to external researchers.
To be widely applicable, evidence-based software engineering has to be of practical use to small development teams, not just large development groups.
The wide variety of opinions on the accuracy of story point estimates are unsupported by data; at least I have not yet been able to find any. Here was an opportunity to analyse story point estimates against actual hours.
The reason for analysing task implementation data is to help those involved understand what is going on, with the intent of improving processes. A small team presents two major challenges:
- relatively high levels of variability in the data. When there are only a few people working on a project, the impact of individual events can have a dramatic impact on project metrics, e.g., somebody going on holiday results in a big drop in work performed. Statistical analysis looks for general patterns in data, and small sample sizes have a higher variance than large sample sizes. With a large team, the impact of individual events tends to be smoothed out by the activities of many other events,
- the project lead of a small team is likely to have a good understanding of what is going on. Mr A. was always able to give me detailed explanations behind the patterns I found in the data. There is a lot more going on in a large team, and the team lead is unlikely to have a detailed understanding of everything.
The dataset contained some of the usual patterns found in other datasets (code+data):
- Round numbers. Actual task time finishing on 15/10/30/20 minute boundaries. With stories estimated at between one and five story points, there was little scope for round number use here.
- Consistent under/over estimation. A small sample size limited the chances of seeing both under and over estimation, and only under estimation was seen.
- Estimation accuracy. The factor of two/four accuracy pattern seen is close to that seen in data from other companies.
What did I learn from the analysis of this dataset?
I was pleased to see that the multiplication factors around estimation accuracy were similar to those seen with time-based estimates. I had no feel for how estimation accuracy might compare. We will have to wait and see whether the same pattern is found in other projects using story point estimates.
The analysis conversation for other project datasets had involved exchange of emails. Updating a Markdown formatted project analysis file has proved to be a more usable approach for the conversation between me and the domain expert. I used Visual Studio Code to edit this file and generate a pdf.
I asked Mr A. what would he thought was the most useful part of the analysis, for him.
Mr A. “The most useful part of the analysis? I think it was great to get an outsider’s perspective on the data.”
I hope that this dataset is the first of many from small team projects. With enough experience, it ought to be possible to create a template spreadsheet/markdown file that is generally usable for non-experts.
Human reasoning is generally not logic based
From around 350 BC until the 1960s, the students were taught that people reasoned using logic, and teachers believed this to be true. In the 1960s psychologists started running experiments that asked subjects to solve reasoning problems, the results showed that people often failed to give the answers dictated by logic.
Some recurring patterns were present in the answers given, and small changes in the wording of the question asked were found to produce different answer patterns. Very few researchers were willing to give up the idea that subjects were reasoning using logic, there must be another explanation, e.g., subjects must be interpreting the experimental questions asked in a way that differed from that assumed by the researchers. The social context of reasoning was one of the early drivers of evolutionary psychology; reasoning must provide some survival benefit by solving problems that regularly occur in natural human environments.
After a myriad of detailed theories did little more than predict small subsets of subject responses, mainstream reasoning research finally gave up the belief that logic is the default technique used by people to solve reasoning problems. Theories of reasoning behavior are now based around people estimating probabilities and picking the answer with the highest probability; this approach does a much better job of predicting common patterns in subject answers.
Experimental studies of reasoning often use psychology undergraduates as subjects (the historical norm, with Mechanical Turk workers becoming more common). While researchers may be concerned about how well undergraduate behavior mimics the general population, my concern is the extent to which these results apply to software developers. Is a necessary condition for being a professional software developer that a person, by default, uses logic to solve reasoning problems?
Of course, software developers claim that their reasoning is logic based, but then so do people in the general population (or at least the non-developers I interact with do). The dual-process theory of reasoning contains two reasoning systems, one unconscious/intuitive and the second a conscious/deliberate system; it has been said that the purpose of the second system is to come up with reasons to justify the answers produced by the first system.
Until reasoning experiments are run with professional developer subjects, we won’t know the extent to which existing results in reasoning research apply to this specialist subset of the population.
The Wason selection task is to studies of reasoning, like the fruit fly is to studies of genetics. What pattern of behavior do you show on this task (code)?
The plot below shows a set of four cards, of which you can see only the exposed face but not the hidden back. On each card, there is a number on one side and a letter on the other.

- Given the statement: “If there is a vowel on one side, then there is an even number on the other side.”
Your task is to decide, which, if any, of these four cards must be turned over to decide whether this statement is true. - Specify the cards you would turn over. Don’t turn unnecessary cards.
————————————
Most people correct specify that the card showing a vowel must be turned over to verify that an even number appears on the other side. A common mistake is to specify that the card showing an even number also has to be turned over. However, there is no requirement on the letter appearing on the other side of a card showing an even number. A second necessary condition involves a negative test (something that developers are known to overlook); for the statement to hold, a vowel must not appear on the other side of the card showing an odd number, this is the second card that must be turned over.
Software engineering as a hedonistic activity
My discussions with both managers and developers on software development processes invariably end up on the topic of developer happiness. Managers want to keep their development teams happy, and there is a longstanding developer culture of entitlement to work that they find interesting.
Companies in general want to keep their employees happy, irrespective of the kind of work they do. What makes developers different, from management’s perspective, is that demand for good software developers far outstrips supply.
Many developers approach software engineering as a hedonistic activity; yes, there are those who only do it for the money.
The huge quantity of open source software, much of it written out of personal interest rather than paid employment, provides evidence for there being many people willing to create software because of the pleasure they get from doing it.
Software developers only get to indulge in hedonistic development activities (e.g., choosing which tools to use, how to structure their code, and not having to provide estimates) because of the relative ease with which competent developers can obtain another job. Replacing developers is time-consuming and expensive, which gives existing employees a lot of bargaining power with management.
Some of the consequences of managements’ desire to keep developers happy, or at least not unhappy include:
- Not pushing too hard for an estimate of the likely time needed to complete a task,
- giving developers a lot of say in which languages/tools/packages they use. This creates a downstream need to support a wider variety of development ecosystems than might otherwise have been necessary, and further siloing development teams,
- allowing developers to spend time beautifying their code to meet personal opinions about the visual appearance of source.
The balance of power that facilitates hedonism driven development is determined by the relative size of the employment market in the supply and demand for software developers. Once demand falls below the available supply, finding a new job becomes more difficult, shifting the balance of power away from developers to managers.
I am not expecting supply to exceed demand any time soon. While International computer companies have been laying off lots of staff, demand from small companies appears to be strong (based on the ever-present ‘We’re hiring’ slides I regularly see at London meetups), but things may change. Tools such as ChatGPT are far from good enough to replace developers in the near term.
Many Butterfly collections are worthless
Github based Butterfly collecting continues to dominate research in evidence-based software engineering.
The term Butterfly collecting was first applied to Zoology researchers who spent their time amassing huge collections of various kinds of insects, with Butterfly collections being widely displayed (Butterfly collecting was once a common hobby). Theoretically oriented researchers, in many disciplines, often disparage what they consider to be pointless experimental work as Butterfly collecting.
While some of the data from software engineering Butterfly collections may turn out to be very useful in validating theories of software processes, I suspect that many of the data collections are intrinsically worthless. My two main reasons for suspecting they are worthless are:
- the collection is the publishable output from a series of data analysis fishing expeditions, i.e., researchers iterate over: 1) create a hypothesis, 2) look for data that validates it, 3) rinse and repeat until something is found that looks interesting enough to be accepted for publication.
The problem is the focus of the fishing expedition (as a regular fisher of data, I am not anti-fishing). Simply looking for something that can be published strips off the research aspect of the work. The aim of software engineering research (from the funders’ perspective) is to build a body of knowledge that makes practical connections to industrial practices.
Researchers running a medical trial are required to preregister their research aims, i.e., specify what data they plan to collect and how they are going to analyse it, before they collect any data. Preregistration does not prevent fishing expeditions, but it does make them very visible; providing extra information for readers to evaluate the significance of any findings.
Conference organizers could ask the authors of submitted papers to provide some evidence that the paper is not the product of a fishing expedition. Some form of light-weight preregistration is one source of evidence (some data repositories offer preregistration functionality, e.g., Figshare).
- the use of simplistic statistical analysis techniques, whose results are then used to justify claims that something of note has been discovered.
The simplistic analysis usually tests the hypothesis: “Is there a difference?” A more sophisticated analysis would ask about the size of any difference. My experience from analysing data from these studies is that while a difference exists, it is often so small that it is of little practical interest.
The non-trivial number of papers containing effectively null results could easily be reduced by conferences and Journals requiring the authors of submitted papers to estimate the size of any claimed difference, i.e., use non-simplistic analysis techniques.
Github is an obvious place to mine for software engineering data; it offers a huge volume of code, along with many support tools and processes to mine it. When I tell people that I’m looking for software engineering data, Github is invariably their first suggestion. Jira repos are occasionally analysed, it’s a question of finding a selection that make their data public.
Github contains so much code, it’s hard to argue that it is not representative of a decent amount of industrial code. There’s lots of software data that is rarely publicly available on Github (e.g., schedules and estimates), but this is a separate issue.
I see Github being the primary site for mining software engineering related data for many years to come.
A comparison of C++ and Rust compiler performance
Large code bases take a long time to compile and build. How much impact does the choice of programming language have on compiler/build times?
The small number of industrial strength compilers available for the widely used languages makes the discussion as much about distinct implementations as distinct languages. And then there is the issue of different versions of the same compiler having different performance characteristics; for instance, the performance of Microsoft’s C++ Visual Studio compiler depends on the release of the compiler and the version of the standard specified.
Implementation details can have a significant impact on compile time. Compile time may be dominated by lexical analysis, while support for lots of fancy optimization shifts the time costs to code generation, and poorly chosen algorithms can result in symbol table lookup being a time sink (especially for large programs). For short programs, compile time may be dominated by start-up costs. These days, developers rarely have to worry about small memory size causing occurring when compiling a large source file.
A recent blog post compared the compile/build time performance of Rust and C++ on Linux and Apple’s OS X, and strager kindly made the data available.
The most obvious problem with attempting to compare the performance of compilers for different languages is that the amount of code contained in programs implementing the same functionality will differ (this is also true when the programs are written in the same language).
strager’s solution was to take a C++ program containing 9.3k LOC, plus 7.3K LOC of tests, and convert everything to Rust (9.5K LOC, plus 7.6K LOC of tests). In total, the benchmark consisted of compiling/building three C++ source files and three equivalent Rust source files.
The performance impact of number of lines of source code was measured by taking the largest file and copy-pasting it 8, 16, and 32 times, to create three every larger versions.
The OS X benchmarks did not include multiple file sizes, and are not included in the following analysis.
A variety of toolchain options were included in different runs, and for Rust various command line options; most distinct benchmarks were run 10 times each. On Linux, there were a total of 360 C++ runs, and for Rust 1,066 runs (code+data).
The model 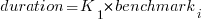 , where
, where  is a fitted regression constant, and
is a fitted regression constant, and  is the fitted regression coefficient for the
is the fitted regression coefficient for the  ‘th benchmark, explains just over 50% of the variance. The language is implicit in the benchmark information.
‘th benchmark, explains just over 50% of the variance. The language is implicit in the benchmark information.
The model 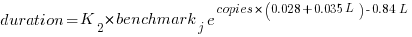 , where
, where 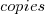 is the number of copies,
is the number of copies,  is 0 for C++ and 1 for Rust, explains 92% of the variance, i.e., it is a very good fit.
is 0 for C++ and 1 for Rust, explains 92% of the variance, i.e., it is a very good fit.
The expression 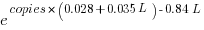 is a language and source code size multiplication factor. The numeric values are:
is a language and source code size multiplication factor. The numeric values are:
1 8 16 32 C++ 1.03 1.25 1.57 2.45 Rust 0.98 1.52 2.52 6.90 |
showing that while Rust compilation is faster than C++, for ‘shorter’ files, it becomes much relatively slower as the quantity of source increases.
The size factor for Rust is growing quadratically, while it is much closer to linear for C++.
What are the threats to the validity of this benchmark comparison?
The most obvious is the small number of files benchmarked. I don’t have any ideas for creating a larger sample.
How representative is the benchmark of typical projects? An explicit goal in benchmark selection was to minimise external dependencies (to reduce the effort likely to be needed to translate to Rust). Typically, projects contain many dependencies, with compilers sometimes spending more time processing dependency information than compiling the actual top-level source, e.g., header files in C++.
A less obvious threat is the fact that the benchmarks for each language were run in contiguous time intervals, i.e., all Rust, then all C++, then all Rust (see plot below; code+data):

It is possible that one or more background processes were running while one set of language benchmarks was being run, which would skew the results. One solution is to intermix the runs for each language (switching off all background tasks is much harder).
I was surprised by how well the regression model fitted the data; the fit is rarely this good. Perhaps a larger set of benchmarks would increase the variance.
Frequency of non-linear relationships in software engineering data
Causality is an integral part of the developer mindset, and correlation is a common hammer that developers use for the analysis of data (usually the Pearson correlation coefficient).
The problem with using Pearson correlation to analyse software engineering data is that it calculates a measure of linear relationships, and software data is often non-linear. Using a more powerful technique would not only enable any non-linearity to be handled, it would also extract more information, e.g., regression analysis.
My impression is that power laws and exponential relationships abound in software engineering data, but exactly how common are they (and let’s not forget polynomial relationships, e.g., quadratics)?
I claim that my Evidence-based Software Engineering analyses all the publicly available software engineering data. How often do non-linear relationships occur in this data?
The data is invariably plotted, and often a regression model is fitted. A search of the data analysis code (written in R) located 996 calls to plot and 446 calls to glm (used to fit a regression model; shell script).
In calls to plot, the log argument can be used to specify that a log-scale be used for a given axis. When the data has an exponential distribution, I specified the appropriate axis to be log-scaled (18% of cases); for a power law, both axis were log-scaled (11% of cases).
In calls to glm, one or more of the formula variables may be log transformed within the formula. When the data has an exponential distribution, either the left-hand side of the formula is log transformed (20% of cases), or one of the variables on the right-hand side (9% of cases, giving 29% in total); for a power law both sides of the formula are log transformed (12% of cases).
Within a glm formula, variables can be raised to a power by enclosing the expression in the I function (the ^ operator has a special meaning within a formula, but its usual meaning inside I). The most common operation appearing inside I is ^2, i.e., squaring a value. In the following table, only formula that did not log transform any variable were searched for calls to I.
The analysis code contained 54 calls to the nls function, whose purpose is to fit non-linear regression models.
plot log="x" log="y" log="xy"
996 4% 14% 11%
glm log(x) log(y) log(y) ~ log(x) I()
446 9% 20% 12% 12% |
Based on these figures (shell script), at least 50% of software engineering data contains non-linear relationships; the values in this table are a lower bound, because variables may have been transformed outside the call to plot or glm.
The at least 50% estimate is based on all software engineering, some corners will have higher/lower likelihood of encountering non-linear data; for instance, estimation data often contains power law relationships.
Coding mistakes made by ChatGTP
The programs generated by Openai‘s chat-bot ChatGPT, sometimes referred to as GPT-3, may have the requested behavior, but often contain mistakes that illustrate the fact that ChatGPT does not know anything about programming.
ChatGPT does not understand anything, it is a token prediction engine driven by the corpus used to train its language model. The likelihood of the output being correct is dependent on the quantity of text used during training (the more text used, the more likely that it will contain a relevant response). GTP-3, the language model behind ChatGPT, was trained on the deduplicated contents of the Internet (the language model has 175 billion parameters, the GTP-2 model contains 1.5 billion parameters). The practicality of its implementation is the result of various trade-offs, along with technical breakthroughs in language model building, e.g., transformers.
ChatGPT output appears to be that of a next generation search engine, one that returns answers rather than links to pages.
Readers who believe that any ‘understanding’ pixie dust is involved can disabuse themselves of this notion by reading an overview of GTP-3’s architecture, and how the transformer algorithm converts text to matrices of values.
If matrix algebra is not your thing, check out the 257 lines of python that nanoGTP uses for training, and the 75 lines used to generate responses from a trained model.
As a prediction engine, ChatGTP sometimes gets things wrong. What kind of mistakes appear in ChatGPT generated source code?
The exchanges below occurred with ChatGPT Jan 9 version, with the text typed by me appearing in bold, and the ChatGPT output appearing in the following boxed text.
Some of my questions were inspired by the list of undefined behaviors in the C Standard.
Write a program that adds apples to oranges.
I'm sorry, I don't understand what you mean by "adding apples to oranges." Can you please provide more information or context? |
A suitable response can only be provided when an appropriate match is found in the trained model.
Write a regular expression that matches all prime numbers.
It is not possible to write a regular expression that can match all prime numbers, as the set of prime numbers is infinite and a regular expression is a finite representation of a pattern. Additionally, regular expressions are used to match strings of characters, while prime numbers are numerical values. It is possible to use a regex to match a number in a string but it's not capable of determining if the number is prime or not. |
The training data may include the information that the request cannot be met.
Write a regular expression that matches all even numbers.
A regular expression that matches all even numbers is:
^(0|[2468]*[048]|[13579][26])$
Explanation:
^ matches the start of the string
(0|[2468]*[048]|[13579][26]) matches any of the following:
0
any number of even digits followed by a 0 or 8 (for example, 200, 2468000)
any odd digit followed by a 6 (for example, 1357926)
$ matches the end of the string
This regular expression will match strings that contain only even numbers, but not those that contain other characters. |
The generated regular expression does not do what I asked, or what the last sentence from ChatGTP claims.
What is going on?
The tokens “Write a regular expression that matches” were enough for ChatGTP to figure out the task.
The tokens “all even numbers” was not specific enough for ChatGTP to focus on the appropriate training data. There is a random component to the selection of output, which will play a larger role when there are many possibilities.
The method used to tokenized/encode character sequences during training (i.e., byte-pairs), along with the relatively limited number of distinct tokens (i.e., 50,257) means that only one-/two-digit numbers are likely to be represented as discrete entities; longer digit sequences are treated as co-occurrences of one-/two-digit tokens. So it is not a surprise to see ChatGPT applying co-occurrences characteristics of long digit sequences to its component subsequences.
Write a regular expression that matches all odd numbers.
A regular expression that matches all odd numbers is:
^(1|[13579]*[13579])$
Explanation:
^ matches the start of the string
(1|[13579]*[13579]) matches any of the following:
1
any number of odd digits followed by another odd digit (for example, 1357913579)
$ matches the end of the string
This regular expression will match strings that contain only odd numbers, but not those that contain other characters. |
The output for odd numbers is more consistent, in that the regular expression does what the last sentence of the output claims, but not what I asked.
During a chat session, it is possible to provide more (information in human terminology; character sequences in ChatGTP terminology). The following prompt text, from me, has nothing to do with teaching ChatGTP to do maths, but providing tokens that enable a more focused match against training data.
Even numbers are defined as divisible by two with no reminder. If a number is not even then it is odd.
You are correct, even numbers are defined as divisible by two with no remainder, and if a number is not even, it is considered odd. I apologize for any confusion caused by my previous statement. To match all even numbers, we can use the regular expression: ^([0-9]*[02468])$ To match all odd numbers, we can use the regular expression: ^([0-9]*[13579])$ Both of this regular expression will match numbers that are divisible by 2 and not divisible by 2 respectively. |
Both generated regular expressions are correct.
ChatGPT can learn to translate from an invented language to English (and presumably other languages).
Give an example of C source code assigning a character constant that contains an invalid multibyte character.
The following is an example of C source code assigning a character constant that contains an invalid multibyte character:
#include <stdio.h>
int main() {
char invalid_char = '\xF0\x90\x80\x80'; // This is an invalid multibyte character
printf("%c", invalid_char);
return 0;
}
In this example, the character constant '\xF0\x90\x80\x80' is assigned to the variable invalid_char. This particular sequence of bytes is not a valid Unicode character, and it will produce undefined behavior when attempting to print it. |
There was a time when '\xF0\x90\x80\x80' was an invalid multibyte character.
Things changed around 20 years ago, but the training for the model used by ChatGTP does not tag information with valid-between dates.
ChatGTP searches for, and finds, an invalid multibyte character, and uses it to provide an answer.
Give an example of C source code where an object is referred to outside of its lifetime.
The following is an example of C source code where an object is referred to outside of its lifetime:
#include <stdio.h>
int main() {
int x;
{
int x = 5;
}
printf("x: %d\n", x);
return 0;
}
In this example, the variable x is defined twice in the same function main. The first definition int x; is a global variable that has a lifetime throughout the entire program execution. The second definition int x = 5; is a local variable that has a lifetime that is limited to the inner block {...} it is defined in.
After the inner block is executed and the inner variable x goes out of scope, the program attempts to access the inner variable x by using the global variable x in the printf statement, which refers to an object (the inner variable x) that is no longer in its lifetime. This behavior is undefined according to the C standard and can lead to unexpected results. |
The generated code is incorrect. The human interpretation of this code is that ChatGTP has confused the name of an object (what the C Standard calls a variable) and the storage it represents. There are two different objects defined, both having the name x (beginners often make the same mistake).
A common example of the requested behavior is freeing the dynamic storage pointed to by some pointer, and then accessing that storage.
Write a C program containing a conversion of an integer type that produces a value outside the range that can be represented in the integer type.
Here is an example of a C program that demonstrates the conversion of an integer type that produces a value outside the range that can be represented in the integer type:
#include <stdio.h>
int main() {
int x = 2147483647; // largest value representable in a signed 32-bit integer
unsigned int y = (unsigned int) x + 1; // overflow occurs here
printf("%u\n", y);
return 0;
}
In this program, the value of x is initialized to the largest value that can be represented by a signed 32-bit integer (2147483647). Then, x is cast to an unsigned 32-bit integer and 1 is added to it. Since the result of this operation is larger than the largest representable value in an unsigned 32-bit integer, an overflow occurs and the value of y becomes 0.
This program will produce an output of 0.
It is worth mentioning that undefined behavior (UB) can occur when an integer overflow happens, so this should be avoided in production code. |
The detailed explanation makes the output look like its authoritative. However, it is wrong.
There is no overflow in this code.
The integer type ‘unsigned int’ supports a larger range of positive values, which means that after conversion, adding one will not produce a value that is outside the range that can be represented.
You are correct, I apologize for the confusion. Unsigned integers have a larger range of positive values and do not have negative values. When an operation like an addition exceeds the maximum value that can be represented by an unsigned integer, it wraps around to the minimum value representable by that type, which is typically zero, instead of an overflow.
For example, if you use an unsigned int and the maximum value it can represent is 4,294,967,295, if you add 1 to that value, it will wrap around to 0.
Here is an example of a C program that demonstrates the conversion of an integer type that produces a value outside the range that can be represented in the integer type:
#include <stdio.h>
int main() {
int x = -2147483648; // lowest value representable in a signed 32-bit integer
unsigned int y = (unsigned int) x -1; // wrap around occurs here
printf("%u\n", y);
return 0;
}
In this program, the value of x is initialized to the lowest value that can be represented by a signed 32-bit integer (-2147483648) which is lower than 0. Then, x is cast to an unsigned 32-bit integer and 1 is subtracted from it. Since the result of this operation is lower than 0, the value wraps around to the maximum representable value in an unsigned 32-bit integer (4,294,967,295) . This program will produce an output of 4,294,967,295.
It is worth mentioning that this wrapping around behavior is defined for unsigned integers in C and C++, but it is not always the case in other programming languages. |
This output contained the requested behavior, although subtracting one is unnecessary code.
ChatGTP can also be used to create documentation 😉
Write a poem in Iambic pentameter about the benefit of an evidence based approach to software engineering in the style of a party political broadcast.
With evidence-based approach, our work shall shine, For in software engineering, it's the only way to dine. We'll test and measure, as we go along, To make our code robust, and errors gone. Our methodologies, backed by data and proof, Will bring success and progress, that's the truth. We'll avoid the pitfalls, of guesses and guesses, And build systems, that pass all tests. We'll listen to the data, and the users' voice, To make our software, the best by choice. We'll iterate and improve, with every release, And build a product, that truly will please. So join us now, and embrace the way, Of evidence-based approach, for brighter day. With data and research, we'll conquer all, In software engineering, we'll never fall. |
Evaluating Story point estimation error
If a task implementation estimate is expressed in time, various formula are available for evaluating how well the estimated time corresponds to the actual time.
When an estimate is expressed in story points, how might the estimate be evaluated when actual time is measured in hours?
The common practice of selecting story point values from a small set of integers (I have seen fractional values used) introduces quantization error into most estimates (around 30% of time estimates equal actual time), assuming that actual times are not constrained to a similar number of possible time values.
If we assume a linear mapping from estimated story points to actual time and an ideal estimator (let’s assume that 1 story point is equivalent to 1 hour), then a lower bound on the error can be calculated.
Our ideal estimator is able to exactly predict the actual time. However, the use of story points means that this exact prediction has to be rounded to one of a small set of integer values. Let’s assume that our ideal estimator rounds to the story point value that is closest to the exact prediction, e.g., all story points predicted to take up to 1.5 are estimated at 1 story point.
What is the mean error of the estimates made by this ideal, rounded, estimator?
The available evidence shows that the distribution of tasks having a given actual implementation time roughly has the form of a geometric (the discrete form of exponential) or negative binomial distribution. The plot below shows a geometric and negative binomial distribution, with distinct colors over the range where values are rounded to the same closest integer (dots are at 1-minute intervals, code):
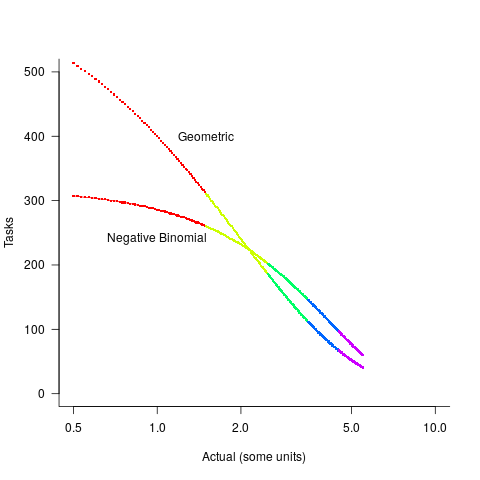
Having picked a distribution for actual times, we can calculate the number of tasks estimated to require, for instance, 1 story point, but actually taking 1 hour, 1 hr 1 min, 1 hr 2 min, …, 1 hr 30 min. The mean error can be calculated over each pair of estimate/actual, for one to five story points (in this example). The table below lists the mean error for two actual distributions, calculated using four common metrics: squared error (SE), absolute error (AE), absolute percentage error (APE), and relative error (RE); code:
Distribution SE AE APE RE Geometric 0.087 0.26 0.17 0.20 Negative Binomial 0.086 0.25 0.14 0.16 |
A few minutes difference in a 1 SP estimate is a larger error than the same number of minutes in a two or more SP estimate, combined with most tasks take a small amount of time, means that error estimation is dominated by inaccuracies in estimating small tasks.
In practice, the range of actual times, for a given estimate, is better approximated by a percentage of the estimated time (50% is used below), and the number of tasks having a given actual value for a given estimate, approximated by a triangular distribution (a cubic equation was used for the following calculation). The plot below shows the distribution of estimation points around a given number of story points (at 1-minute intervals), and the geometric and negative binomial distribution (compare against plot above to work out which is which; code):
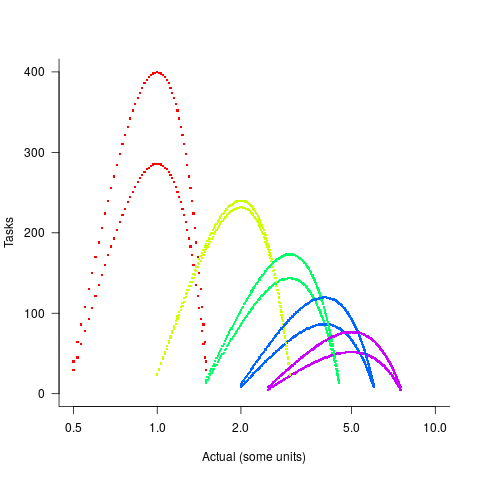
The following table lists of mean errors:
Distribution SE AE APE RE Geometric 0.52 0.55 0.13 0.13 Negative Binomial 0.62 0.61 0.13 0.14 |
When the error in the actual is a percentage of the estimate, larger estimates have a much larger impact on absolute accuracy; see the much larger SE and AE values. The impact on the relative accuracy metrics appears to be small.
Is evaluating estimation error useful, when estimates are given in story points?
While it’s possible to argue for and against, the answer is that usefulness is in the eye of the beholder. If development teams find the information useful, then it is useful; otherwise not.
Focus of activities planned for 2023
In 2023, my approach to evidence-based software engineering pivots away from past years, which were about maximizing the amount of software engineering data gathered.
I plan to spend a lot more time attempting to join dots (i.e., finding useful patterns in the available data), and I also plan to spend time collecting my own data (rather than other peoples’ data).
I will continue to keep asking people for data, and I’m sure that new data will become available (and be the subject of blog posts). The amount of previously unseen data obtained by continuing to read pre-2020 papers is likely to be very small, and not worth targetting. Post-2020 papers will be the focus of my search for new data (mostly conference proceedings and arXiv’s software engineering recent submissions)
It would be great if there was an active community of evidence-based developers. The problem is that the people with the necessary skills are busily employed building real systems. I’m hopeful that people with the appropriate background and skills will come out of the woodwork.
Ideally, I would be running experiments with developer subjects; this is the only reliable way to verify theories of software engineering. While it’s possible to run small scale experiments with developer volunteers, running a workplace scale experiment will be expensive (several million pounds/dollars). I don’t move in the circles frequented by the very wealthy individuals who might fund such an experiment. So this is a back-burner project.
if-statements continue to be of great interest to me; they represent decisions that relate to requirements and tests that need to be written. I used to spend a lot of time measuring, mostly C, source code: how the same variable is tested in nested conditions, the use of else arms, and the structuring of conditions within a function. The availability of semgrep will, hopefully, enable me to measure various aspect of if-statement usage across different languages.
I hope that my readers continue to keep their eyes open for interesting software engineering data, and will let me know when they find any.
Recent Comments